A B C D E F 

B.  Curvature

So how can space, or spacetime, have a curvature?  What does that mean?  There doesn't seem to be anything obviously curved about either of them.

It helps here to think about some things that obviously are curved, and see what we can learn from them that we might apply to spacetime.  Consider a basketball.  Its surface is clearly not flat like a sheet of paper or a table top.  We could make a sheet of paper more curved, like the basketball, by wrapping the sheet of paper around the basketball.  When we do, we take the length and width of the sheet of paper and fit them to the basketball's surface by bending the paper's length and width through a third dimension.  This third dimension is outside the paper surface's original two.

Likewise, one way to give a curvature to a three- or four-dimensional continuum like space or spacetime is to bend it through some additional dimensions, outside the continuum's three or four own dimensions.

This is easy to say, but imagining such a curvature presents a problem.  We live in the three dimensions of space and one dimension of time, and we lack experience with moving around in additional similar dimensions.  It's not obvious how to visualize extra dimensions in which spacetime might be curved.

We can, however, understand our situation better by imagining an analogous problem for a two-dimensional being, living in two-dimensional space, who can't imagine a third spatial dimension.  As we see in Figure 1, the space wherein he lives might be curved through the third dimension, or even through fourth, fifth, or additional dimensions, but he wouldn't be any more able than we are to imagine extra dimensions outside his space.

On the other hand, if he has the right tools, he could determine whether his two-dimensional space were curved, even though he couldn't visualize the curvature directly.  One way to do it would involve his making and surveying a circle.

Suppose this two dimensional person lived in a flat place.  He could pick a point in this place to be the center of a circle, and make out all the points that were some distance x from this center to be the circle's circumference.  If he then measured the length of this circumference, and compared it to the length of one of the circle's diameters, he would find the circumference to be about 3.14159265... times as long as the diameter-the familiar ratio π.

But if he tried the same thing in a curved two-dimensional space, he would get a different result.  Suppose his two-dimensional space were the surface of a very large sphere.  The diameter of a circle would be slightly longer than it would in a plane, since the sphere's curvature would make the circle's diameter bulge outward a little into the third dimension.  Thus the circle's circumference would be less than times as long as its diameter.  The bigger the circle, the smaller its circumference-to-diameter ratio would be.  With a different kind of curvature, our two-dimensional man would even find an opposite result.  Suppose our man lived in, rather than a spherical surface, a surface in the shape of a horse's saddle, or the shape of one of those potato snacks that comes in a tall can.  A circle mapped out on a surface like that would have a circumference more than π times longer than its diameter, the ratio being greater for a larger circle.  In either case, our two-dimensional surveyor could detect the curvature of his space, and even determine the general nature and degree of its curvature, just by making appropriate measurements within his space's own two dimensions.  To do so, he would not have to leave his two-dimensional space, or even be able to imagine what its curvature would look like in any extra dimensions.

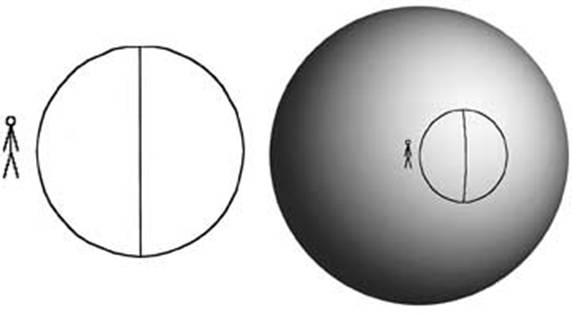
Figure 1.  A circle in a flat plane has a circumference-to-diameter ratio of π (3.14159265...).  On a sphere, a circle's circumference is less than π times the length of a diameter.  A two-dimensional surveyor could thus detect the curvature of his world even if he couldn't see the third dimension.

We can do something similar in our four-dimensional spacetime.  By making appropriate measurements within spacetime itself, we should be able to tell whether spacetime has a curvature.  The measurements need not be confined to circles.  Einstein's general relativity theory suggests many different ways that curvature should show up in physical phenomena.  Each of these ways in fact provides a different test of Einstein's theory.  General relativity not only says that spacetime can be curved, but that it will curve in certain ways, by certain amounts, under certain circumstances.

The curvature may be as complex as the circumstances that determine it.  The connection between the circumstances and the curvature was not trivial for Einstein to discover; he had plenty of information to consider as he figured out the law relating the two, and he didn't understand everything all at once.  Nonetheless, the law he eventually formulated is a simple one, as is its relation to gravity.     (.....continued)

A B C D E F